Oxford University Cave ClubCave Research Group publication 141961 Oxford University Expedition to Northern Spain |
1961 Expedition Report (CRG 14) |
Any expedition contemplating scientific work abroad is wise to make sure that its members are fully conversant with the equipment before leaving the shores of Britain. Preparations were made for geoelectric survey in Spain during a practice survey at Bull Pot Farm, Casterton Fell, Westmorland in July 1961.
Many caves and potholes were explored and surveyed to as high a degree of accuracy as possible with the equipment and time available to the expedition. A surface survey was also carried out by levelling for some potholes, as a result of which the depth of the passage below the surface was known to a fair degree of accuracy at various points. These depths were compared with results calculated from geoelectric depth surveys using the 'expanding electrode' method, and results for Pozo Palomeru (P1) are given in Appendix C.
The Nash and Thompson Geophysical Tellohm (Plate 4) was used in Spain and operates by means of the 'null' principle. The A.C. voltage is generated by a reversing relay (110 c/s) driven from a 30 volt dry cell. A second set of contacts on the relay provides synchronous rectification of the difference between the voltage across the secondary of a transformer (driven from the exciting current) and the potential probe voltage, so that a sensitive D.C. galvanometer can be used as a null detector. Since the current flowing in the potential circuit at the null balance point is zero, potential probe resistance has no effect on the accuracy of the results. Operation of the meter is extremely simple and quick, employing a direct-reading linear-scaled potentiometer. The galvanometer needle moves for convenience in the same direction as the range switch and potentiometer. A probe selector box may be attached to the instrument by means of which various parts of the circuit may be checked before readings are taken. The complete instrument is fairly light and compact and may be carried like a suitcase. This instrument may be highly recommended for the detection of caves.
A frame was constructed from Dexion slotted angle to carry four 300 ft. reels of wire, and straps were attached to enable the frame to be carried as a rucksack. The frame was padded in suitable places with foam rubber. Upon arrival at the survey site, the frame was placed with the reels uppermost near to the instrument. The wire was brought out at the centre of each reel, and terminated with a small crocodile clip. It was then an easy matter to connect these clips to four flying leads attached to the P and C terminals of the instrument. All ancillary equipment was carried in another rucksack. It was found that the method had the disadvantage that the reels could not be unwound without disconnecting the clips, but in practice this was not a serious limitation. Two extra reels, each of 300 ft, were used when necessary for the extension of the current leads. Electrodes were of mild steel, of half-inch diameter, and case hardened. The cranked, steel electrodes supplied by Martin-Clark Instruments, were found most convenient, as these can be inserted without the use of a hammer and without bending down, it being only necessary to press them into the soil with the foot.
Connections were made to the electrodes by strong battery clips and single-core cable. Connections by this method were always found to be good, but a disadvantage is that the clips tend to be caught up in the surface vegetation as the wires are moved. Electrode extractors, consisting of flat metal bars drilled at the ends to provide leverage around the shanks of the electrodes, were found to be very satisfactory, except in those cases where the electrodes became burred at the top, thus preventing the insertion of the electrode in the holes of the extractors. Wooden tent pegs were used to mark survey stations, and metallic tapes were used to measure the traverses, whilst canes were used to mark anomalies detected in the field.
The surveyors were very impressed by the way that the instruments stood up to rough handling. They were often shaken roughly during transport over the limestone terrain with clint surfaces, slippery rocks, scree, scrub and small trees, yet continued to function perfectly. The instruments first of all had to stand up to road transport.
The Wenner four-electrode method employing equal separation of electrodes was used for all step-traverses over known and conjectured caves. In some depth surveys by the 'expanding electrode' method, however, the ratio of potential electrode to current electrode separation was increased from the normal value of 1/3 (0.33) for the Wenner method (see notes on theory of application: Appendix A). A maximum separation of 200 feet was employed in step traverses, although the average separation was 20 feet. Depth surveys were carried out with a separation of the current electrodes of up to 600 feet.
Results for the surveys carried out over Pozo Palomeru are given in Appendix C. Marshy conditions short circuit the current and features are masked under these circumstances. This unfortunately was the case for a survey carried out across the floor of the polje Las Reblagas. An attempt was made to locate the connection between the Ercina sink S4 and the Las Reblagas resurgence R2, proved by hydrological testing, but resulted in failure because of the inadequate depth reached by the depth survey.
The method may be regarded as a useful way of confirming the presence of a passage at depth in limestone, and of finding new caves. The accuracy is, however, limited, and the restriction of the method to suitably flat stretches of ground without rock, and of adequate length, is a severe handicap among the clint surfaces which are likely to occur over many possible sites of caverns.
In cases where the separation of the current electrodes cannot be increased because of rocky ground, etc., it may be possible to reach a greater depth by increasing the ratio of the potential electrode to current electrode separation. Some consequences of this procedure are mentioned in Appendix A.
The normal field procedure for a step-traverse using the Wenner configuration is to place the line of the traverse perpendicular to the general line of the cave, if known. This is termed the 'end-on' method of traverse, and gives reversed readings over anomalies, i.e. the cave anomaly appears as a trough with a peak on either side of it when a resistivity-distance graph is plotted from the results, an effect caused by the successive passage of electrodes above the cave. An alternative method of operation, the 'broadside' method of traverse, gives anomalies in the correct sense, but is less convenient to carry out than the 'end-on' method, since all four electrodes must be moved together. Hence, if end-on traverses are used, the surveyor must always allow for the reversed readings, and interpretation of curves is only learnt by experience.
The problem of locating caves by resistivity methods can thus be seen to be not too difficult, the air space in the cave having for practical purposes an infinite resistivity, and the problem thereby approximates to a 'two-layer' situation. A large number of calculated curves have been published, and these may be used for the production of more accurate and reliable results. By comparing the type curves for the calculated 'two-layer' situations with those obtained in the field, it may be possible to obtain depth values from the nearest calculated curve to a high degree of accuracy. For less accurate determinations the position of the point of inflection on a depth survey curve may be used to calculate the depth of an anomaly.
The resistivity method of geophysical surveying can have an important role to play in the support of a caving programme, and can provide corroboratory evidence of the course of a cave where needed. It is therefore worthy of attention by those intending to undertake speleological research, whether abroad or in Britain.
John D. Wilcock, Kidsgrove July 1964.
Bibliography
The Wenner method of geoelectric survey is the most suitable for long step-traverses, as it does not require the replacement of electrodes at each reading; each electrode serves as (current electrode) C1, (potential electrode) P1, P2 and C2 as the step-traverse progresses. The equipment causes an electric current (A.C.) to be passed through the earth between the two outer electrodes, C1 and C2, and the voltage is measured between the two inner electrodes P1 and P2. Let the distance between the current electrodes be 2a and that between the potential electrodes 2b. The ratio b/a is called α in the analysis below; for the Wenner method, α is always equal to 1/3 (0.33). The instrument converts the current and voltage readings into a resistance reading, R ohms, and from this the specific resistance, or resistivity of the earth in the vicinity can be found. The ratio of the resistivity r to the resistance R is given by the expression:
![]()
For the Wenner method ( α = 0.33) this expression reduces to:
ρ = 2πdR
where d is the separation of the electrodes (d = a - b = 2a/3). Thus the Wenner method has the advantage of a direct proportionality between the resistivity and resistance for a number of readings, and for convenience resistance, directly read from the instrument, may be plotted on the curves instead of resistivity.
Some authors suggest that the depth of penetration of the survey is of the order of d, the separation of the electrodes. More accurate analysis, however, gives the following expression for the depth h:
![]()
The work of the expedition seems to confirm this second, more accurate formula. Large values of d were often used to get penetration of 100 ft or more, and at these depths the accuracy of the instrument was sufficient to distinguish between the values d and a Ö (0.33) = 0. 58a = 0. 86d.
In some depth surveys the separation of the current electrodes may perhaps not be increased beyond a certain value because of rocky ground, etc. Under these circumstances it may be possible to reach a greater depth by increasing the value of α , i.e. by increasing the separation of the potential electrodes without moving the current electrodes. Unfortunately if α is altered during a survey the resistance measured on the instrument is no longer proportional to the resistivity, since
![]()
A quick method of calculating ρ in the field, given R and α , is needed, and a slide rule may be modified to this end as follows. Values of the expression:
![]()
are calculated for all possible values of α . The corresponding values of α are then marked by pencil or other means along the top (fixed) scale of the slide rule at the numerical positions given by the calculated values of the above expression. If now the value of a , half the distance between the current electrodes, is set on the upper sliding scale opposite the value of α currently in use, the value of ρ may be read off on the upper sliding scale opposite the resistance reading R on the upper fixed scale. This method has proved a useful way of calculating values of ρ rapidly in the field. Values of α and the calculated value of ρ are recorded in the notebook for each reading. It is then a simple matter to calculate the depth reached ( aÖα ) by further use of the slide rule.
For 'two-layer' problems theoretical curves may be plotted using the expression:
![]()
where
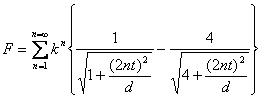
and ρ1 = resistivity of upper layer; ρd = 2πdR; k = ( ρ2 - ρ1 ) / ( ρ2 + ρ1 ); t = depth below surface of junction between layers; ρ2 = resistivity of lower layer.
For the cave problem, ρ2 may be taken as near-infinite, and k is effectively unity.
The results for the geoelectric survey over P1 were compared with the surface survey over the explored passages, from which the depth of the cave at various points could be calculated with a fair degree of accuracy. A surface dry valley conveniently lies above the passage in one place, and a step traverse here gave an anomaly agreeing well with the surface and underground surveys; a depth survey at this point gave a point of inflection at an electrode spacing of 80 ft, with a resistivity of 6,300 ohm-ft, many times the normal value for limestone. The surface survey (see Figure 3) predicts a maximum depth for the passage of 60 ft, and in some places a depth of only 20 ft. Hence the electrode spacing is not equal to the depth of the cave, and the value a Öα , in this case equal to 69 ft, is in better agreement.
Another survey across the dry valley beyond the furthest point explored underground indicated the presence of a cave, which may be the extension of the known passages. A depth survey indicated a cave at about 35 ft below the surface.
The following table gives corresponding values of d and a Öα for a number of electrode separations:
|
b |
a |
d (= a -
b) |
a
Öα |
|
|
|
|
|
|
5 |
15 |
10 |
8.7 |
|
10 |
30 |
20 |
17.3 |
|
15 |
45 |
30 |
25.9 |
|
20 |
60 |
40 |
34.6 |
|
25 |
75 |
50 |
43.3 |
|
30 |
90 |
60 |
51.9 |
|
35 |
105 |
70 |
60.6 |
|
40 |
120 |
80 |
69.3 |
|
45 |
135 |
90 |
77.9 |
|
50 |
150 |
100 |
86.6 |
Manufacturers: Nash and Thompson Limited, Oakcroft Road, Chessington, Surrey.
Dimensions: 14. 25 x 11.125 x 5 inches (36 x 28 x 13 cm.)
Weight: 16. 5 Ib (7. 5 Kg.) less batteries; may be carried as a suitcase.
Ranges: 0 - 0.3, 0 - 1, 0 - 3, 0 - 10, 0 - 100, 0 - 1,000, 0 - 10, 000 ohms.
Scale: Linear, direct reading on potentiometer.
Principle of operation: Null method. A.C. voltage generated by reversing relay driven from dry battery power supply. A second set of contacts on the relay provides synchronous rectification of the difference between the potential circuit e.m.f. and a transformer secondary output voltage, regulated by the potentiometer. Sensitive galvanometer used as null detector.
Frequency of operation: 110 c/s. nominal.
Operating voltage: 150 volts.
Power supply: Five AD4 cells (total of 30 volts).
Advantages: Extreme simplicity of operation; balance may be obtained rapidly; compact; housed in laminated plastic case with metal reinforcing corners; leakproof battery housing; range selector switch and galvanometer needle move in same direction as potentiometer dial during operation.
Accessory: Probe Selector Box, mounted on top of battery housing, enables measurements of the individual probe resistances to be taken for checking purposes.